Maths Sample Paper Class 10 2024
Maths Sample Paper Class 10 2024
CLASS – X (SESSION: 2024-25)
SUBJECT – MATHEMATICS (STANDARD)
Time Allowed: 3 Hrs Maximum Marks:80
General Instructions:
- This Question Paper has 5 Sections A-E
- Section A has 20 MCQs carrying 1 mark each.
- Section B has 5 questions carrying 02 marks each.
- Section C has 6 questions carrying 03 marks each.
- Section D has 4 questions carrying 05 marks each.
- Section E has 3 case based integrated units of assessment (04 marks each) with sub- parts of the values of 1, 1 and 2 marks each respectively.
- All Questions are compulsory. However, an internal choice in 2 Questions of 5 marks, 2 Questions of 3 marks and 2 Questions of 2 marks has been provided. An internal choice has been provided in the 2 marks questions of Section E
- Draw neat figures wherever required. Take π =22/7 wherever required if not stated.
SECTION-A
Section A consists of 20 questions of 1 mark each.
- If p and q are two different positive integers, p=18a2b4 and q=20a4b2,where a and b are prime numbers. Then LCM of p and q is : a) 2a2b4 b)180a4b2 c) 12a4b4 d) 180a4b4
- The graph of a quadratic polynomial p(x) passes through the points (-4,0) , (0,-20), (4,-20) and (7,0). Zeroes of the polynomial are a) -4,0 b) 4,7 c) -3 ,20 d) -4,7
- A quadratic polynomial, whose zeroes are -4 and -5, is
(a) x²-9x + 20 (b) x² + 9x + 20
(c) x²-9x- 20 (d) x² + 9x- 20 - If a pair of linear equations is consistent, then the lines will be
(a) always coincident (b) parallel
(c) always intersecting (d) intersecting or coincident - The discriminant of the equation (x +1)3 = 4 -x + x3 is
(a) 52 (b) 53
(c) 64 (d) 72 - The (n – 1)th term of the A.P. 7,12,17, 22,……. is
(a) 5n + 2 (b) 5n + 3 (c) 5n – 5 (d) 5n – 3
- AD is the median of ∆ABC with vertices A(5.-6) ,B(6,4) C(0,0). Length AD is equal to
(a) √68units b) 2√15units c) √101units d) 10 units
8. A line intersects the y-axis and x-axis at the points P and Q, respectively. If (2, –5) is the mid point of PQ, then the coordinates of P and Q are, respectively.
(a) (0, – 5) and (2, 0) (b) (0, 10) and (– 4, 0)
(c) (0, 4) and (– 10, 0) (d) (0, – 10) and (4, 0)
9. (Sec A + Tan A)(1-SinA) equals
a) Sec A b) Sin A c) Cosec A d) Cos A
10 . If cos (α+ β)=0 , then value of cos (α+ β)/2 is equal to
(a) 1/2 (b) 1/√2 ( c) √2 (d) 0
11. If two tangents inclined at an angle 600 are drawn to a circle of radius 3cm , then length of each tangent is
a) (3√3)/2 cm b) 3cm c) 6cm d) 3√3cm
12. The volumes of two spheres are in the ratio 27 : 8. The ratio of their curved surface areas is:
(a) 9 : 4 (b) 4 : 9 (c) 3 : 2 (d) 2 : 3
13. AB is a chord of the circle and AOC is its diameter such that angle ∠ACB = 50°. If AT is the tangent to the circle at the point A, then ∠BAT is equal to

(a) 65° (b) 60° (c) 50° (d) 40°
14. The relationship between mean, median and mode is
(a) mode = median – 2 mean (b) mode = 3 median – 2 mean
(c) mode = 2 median – 3 mean (d) mode = median – mean
15. Ajay drew a card at random from a deck of cards. What is the probability of a card drawn to be a red card or a black card and what can we say about that event ?
(a) 0 and it is a sure event (b) 1 and it is a sure event
(c) 0 and it is an impossible event (d) 1 and it is an impossible event
16. The probability that a non-leap year selected at random will contain 53 Sundays is:
(a) 1/7 (b) 2/7 (c) 3/7 (d) 5/7
17. If a parallelogram circumscribes a circle, then the parallelogram is a a) Trapezium b) square c) rectangle d) rhombus 18. If the class marks of a continuous frequency distribution are 22, 30, 38, 46, 54, 62, then the class corresponding to the class mark 46 is a) 41.5 – 49.5 b) 42 – 50 c) 41 – 49 d) 41 – 50
Direction for questions 19 & 20: In question numbers 19 and 20, a statement of Assertion (A) is followed by a statement of Reason (R). Choose the correct option
- Both Assertion (A) and Reason (R) are true and Reason (R) is correct explanation of Assertion (A).
- Both Assertion (A) and Reason (R) are true but Reason (R) is not the correct explanation of Assertion (A).
- Assertion (A) is true but Reason (R) is false.
- Assertion (A) is false but Reason (R) is true. 19. Assertion (A): The length of the minute hand of a clock is 7 cm. Then the area swept by the minute hand in 5 minutes is 77/6 cm2.
Reason (R): The length of an arc of a sector of angle θ and radius r is given by

20. Assertion(A): Total surface area of a cylinder having radius of the base 14cm and height 30cm is 3872 cm2
Reason (R) : If r be the radius and h be the height of the cylinder, then total surface area of cylinder is 2Πrh + 2Πr2
SECTION-B
Section B consists of 5 questions of 2 marks each
21. Explain why 3 x 5 x 7 x 9 x 11 + 11 is a composite number ?
OR
Find : HCF(345, 405, 270).
22. Find the ratio in which the line segment joining the points A(-3,10) , B(6,-8) is divided by the point P(-1,6).
23. If the points A(6,1), B(8,2) , C(9,4) and D(p,3) are the vertices of a parallelogram, taken in order, find the value of p.
24. If ∆ ABC ~ ∆RPQ, AB = 3 cm, BC = 5 cm, AC = 6 cm, RP = 6 cm and PQ = 10 cm, then find QR.
OR
If D is any point on the side BC of a ∆ABC such that, angle ADC = angle BAC Prove that BC/CA =CA/CD.
25. Two dice are thrown simultaneously. Find the probability of getting a doublet.
SECTION-C
Section C consists of 6 questions of 3 marks each.
26. Prove that √3 is irrational.
27. If α and β are the two zeroes of the polynomial 25p2 – 15p + 2, find a quadratic polynomial whose zeroes are 1/2α and 1/2β .
OR
If sum of the squares of zeroes of the quadratic polynomial x2– 8x +k is 40, find the value of k.
28. Which are two consecutive odd positive integers, sum of whose squares is 290?
29. Prove that if a line is drawn parallel to one side of a triangle, then it divides the other two sides in the same ratio.
OR
Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of triangle PQR. Show that ∆ABC ~ ∆PQR.
30. If Sec A = x + 1/4x , then prove that sec A + tan A = 2x or 1/2x
31. A chord of a circle of radius 10 cm subtend a right angle at the centre of the circle. Find the area of the corresponding minor segment and major sector. ( use Π=3.14)
SECTION-D
Section D consists of 4 questions of 5 marks each
32. The monthly income of A and B are in the ratio of 9: 7. If their monthly expenditures are in the ratio 4:3. If each saves Rs 1600 per month. Find the monthly incomes of each?
OR
The sum of a 2 digit number and the number obtained by reversing the digits is 110. If 10 is subtracted from the first number, the new number is 4 more than 5 times the sum of the digits in the first number. Find the first number.
33. A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively (see figure). Find the sides AB and AC.

34. A 1.5 m tall boy is standing at some distance from a 30 m tall building. The angle of elevation from his eyes to the top of the building increases from 30° to 60° as he walks towards the building. Find the distance he walked towards the building.
OR
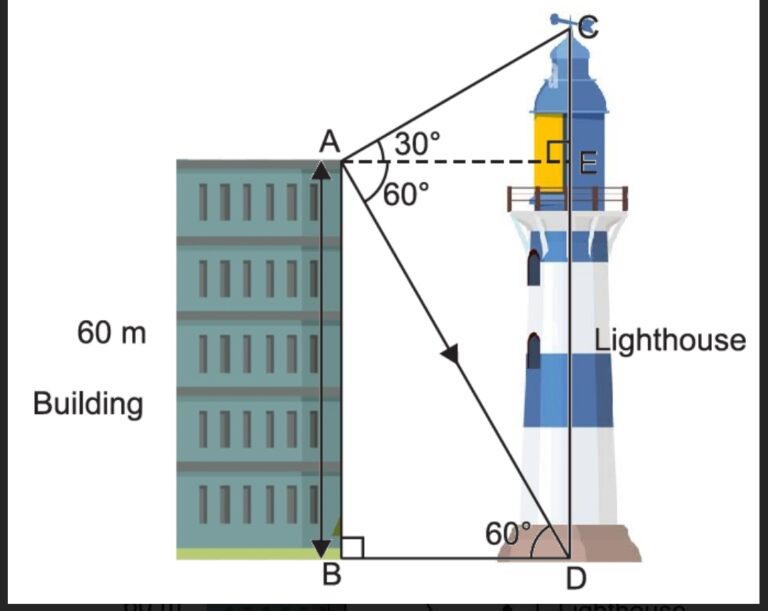
The angle of elevation of a cloud from a point 60m above a lake is 300 and the angle of depression of the reflection of the cloud in the lake is 600. Find the height of the cloud.
35. Find mean and median of the following data
| Class intervals | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 |
| Frequency | 7 | 14 | 13 | 12 | 20 | 11 | 15 | 8 |
SECTION-E
This section comprises 3 case study based questions of 4 marks each
Case Study – 1
36. Lumber is a significant natural resource that contributes jobs to the US economy. Lumber companies source their raw materials from privately-managed or government-leased forests. In order to process tree wood into usable lumber, this raw material is transported to lumber mills, where it is cut to different sizes. Lumber is primarily used by the construction industry, though it can also be used to produce furniture, paper and pulp, and composites such as plywood. A lumber company stacks 200 logs in the manner as shown in the attachment.
20 logs in the bottom row, 19 in the next row, 18 in the row next to it and so on.
Based on the above information answer the following questions:

(i) Find the number of rows in which 200 logs are stacked.
(ii) Find the number of logs in the top row.
(iii) a ) What is the difference between the number of logs in 10th and 6th row ?
OR
(iii) (b) How many total logs in middle rows ?
Case Study – 2
37. Tamper-proof tetra-packed milk guarantees both freshness and security. This milk ensures uncompromised quality, preserving the nutritional values within and making it a reliable choice for health-conscious individuals. 500 ml milk is packed in a cuboidal container of dimensions 15 cm x 8 cm x 5 cm. These milk packets are then packed in cuboidal cartons of dimensions 30 cm x 32 cm x 15 cm. Based on the above given information, answer the following questions

(i) Find the volume of the cuboidal carton.
(ii) How much milk can the cup (as shown in the figure) hold?
(iii) (a) Find the total surface area of a milk packet.
OR
(b) How many milk packets can be filled in a carton ?
Case study – 3
38. Triangle is a very popular shape used in interior designing. The picture given shows a cabinet designed by a famous interior designer.

Here the largest triangle is represented by △ABC and smallest one with shelf is represented by △DEF. PQ is parallel to EF.
(i) Show that △ DPQ ~ △ DEF.
(ii) If DP = 50 cm and PE = 70 cm then find PQ/EF.
(iii) (a) If 2AB = 5DE and △ ABC ~ △ DEF then show that ratio of the perimeters of △ ABC and △ DEF is constant.
OR
(b) If AM and DN are medians of triangles ABC and DEF respectively then prove that △ABM ~ △DEN.
Check answers and solutions of Maths Sample Paper Class 10 2024


CLICK THE LINK FOR OUR YOUTUBE CHANNEL CLASS 10 VIDEOES
https://www.youtube.com/watch?v=cZNRKw-2-x8&list=PL2uPMjJCHErQZn29FbNE80j5HF8FH8Nl6
Other posts on website https://sharmatutorial.in